
Graphene nanoribbons (GNRs, also called nano-graphene ribbons or nano-graphite ribbons) are strips of graphene with width less than 100 nm. Graphene ribbons were introduced as a theoretical model by Mitsutaka Fujita and coauthors to examine the edge and nanoscale size effect in graphene.[2][3][4]
Production
Nanotomy
Large quantities of width-controlled GNRs can be produced via graphite nanotomy,[5] where applying a sharp diamond knife on graphite produces graphite nanoblocks, which can then be exfoliated to produce GNRs as shown by Vikas Berry. GNRs can also be produced by "unzipping" or axially cutting nanotubes.[6] In one such method multi-walled carbon nanotubes were unzipped in solution by action of potassium permanganate and sulfuric acid.[7] In another method GNRs were produced by plasma etching of nanotubes partly embedded in a polymer film.[8] More recently, graphene nanoribbons were grown onto silicon carbide (SiC) substrates using ion implantation followed by vacuum or laser annealing.[9][10][11] The latter technique allows any pattern to be written on SiC substrates with 5 nm precision.[12]
Epitaxy
GNRs were grown on the edges of three-dimensional structures etched into silicon carbide wafers. When the wafers are heated to approximately 1,000 °C (1,270 K; 1,830 °F), silicon is preferentially driven off along the edges, forming nanoribbons whose structure is determined by the pattern of the three-dimensional surface. The ribbons had perfectly smooth edges, annealed by the fabrication process. Electron mobility measurements surpassing one million correspond to a sheet resistance of one ohm per square — two orders of magnitude lower than in two-dimensional graphene.[13]
Chemical vapor deposition
Nanoribbons narrower than 10 nm grown on a germanium wafer act like semiconductors, exhibiting a band gap. Inside a reaction chamber, using chemical vapor deposition, methane is used to deposit hydrocarbons on the wafer surface, where they react with each other to produce long, smooth-edged ribbons. The ribbons were used to create prototype transistors.[14] At a very slow growth rate, the graphene crystals naturally grow into long nanoribbons on a specific germanium crystal facet. By controlling the growth rate and growth time, the researchers achieved control over the nanoribbon width.[15]
Recently, researchers from SIMIT (Shanghai Institute of Microsystem and Information Technology, Chinese Academy of Sciences) reported on a strategy to grow graphene nanoribbons with controlled widths and smooth edges directly onto dielectric hexagonal boron nitride (h-BN) substrates.[16] The team use nickel nanoparticles to etch monolayer-deep, nanometre-wide trenches into h-BN, and subsequently fill them with graphene using chemical vapour deposition. Modifying the etching parameters allows the width of the trench to be tuned to less than 10 nm, and the resulting sub-10-nm ribbons display bandgaps of almost 0.5 eV. Integrating these nanoribbons into field effect transistor devices reveals on–off ratios of greater than 104 at room temperature, as well as high carrier mobilities of ~750 cm2 V−1 s−1.
Multistep nanoribbon synthesis
A bottom-up approach was investigated.[17][18] In 2017 dry contact transfer was used to press a fiberglass applicator coated with a powder of atomically precise graphene nanoribbons on a hydrogen-passivated Si(100) surface under vacuum. 80 of 115 GNRs visibly obscured the substrate lattice with an average apparent height of 0.30 nm. The GNRs do not align to the Si lattice, indicating a weak coupling. The average bandgap over 21 GNRs was 2.85 eV with a standard deviation of 0.13 eV.[19]
The method unintentionally overlapped some nanoribbons, allowing the study of multilayer GNRs. Such overlaps could be formed deliberately by manipulation with a scanning tunneling microscope. Hydrogen depassivation left no band-gap. Covalent bonds between the Si surface and the GNR leads to metallic behavior. The Si surface atoms move outward, and the GNR changes from flat to distorted, with some C atoms moving in toward the Si surface.[19]
Electronic structure
The electronic states of GNRs largely depend on the edge structures (armchair or zigzag). In zigzag edges each successive edge segment is at the opposite angle to the previous. In armchair edges, each pair of segments is a 120/-120 degree rotation of the prior pair. The animation below provides a visualization explanation of both. Zigzag edges provide the edge localized state with non-bonding molecular orbitals near the Fermi energy. They are expected to have large changes in optical and electronic properties from quantization.[20]
Calculations based on tight binding theory predict that zigzag GNRs are always metallic while armchairs can be either metallic or semiconducting, depending on their width.[20] However, density functional theory (DFT) calculations show that armchair nanoribbons are semiconducting with an energy gap scaling with the inverse of the GNR width.[21] Experiments verified that energy gaps increase with decreasing GNR width.[22] Graphene nanoribbons with controlled edge orientation have been fabricated by scanning tunneling microscope (STM) lithography.[23] Energy gaps up to 0.5 eV in a 2.5 nm wide armchair ribbon were reported.
Armchair nanoribbons are metallic or semiconducting and present spin polarized edges. Their gap opens thanks to an unusual antiferromagnetic coupling between the magnetic moments at opposite edge carbon atoms. This gap size is inversely proportional to the ribbon width[20][24][25] and its behavior can be traced back to the spatial distribution properties of edge-state wave functions, and the mostly local character of the exchange interaction that originates the spin polarization. Therefore, the quantum confinement, inter-edge superexchange, and intra-edge direct exchange interactions in zigzag GNR are important for its magnetism and band gap. The edge magnetic moment and band gap of zigzag GNR are reversely proportional to the electron/hole concentration and they can be controlled by alkaline adatoms.[26]
Their 2D structure, high electrical and thermal conductivity and low noise also make GNRs a possible alternative to copper for integrated circuit interconnects. Research is exploring the creation of quantum dots by changing the width of GNRs at select points along the ribbon, creating quantum confinement.[27][20] Heterojunctions inside single graphene nanoribbons have been realized, among which structures that have been shown to function as tunnel barriers.
Graphene nanoribbons possess semiconductive properties and may be a technological alternative to silicon semiconductors[28] capable of sustaining microprocessor clock speeds in the vicinity of 1 THz[29] field-effect transistors less than 10 nm wide have been created with GNR – "GNRFETs" – with an Ion/Ioff ratio >106 at room temperature.[30][31]
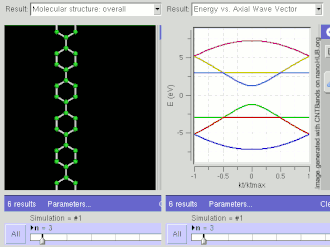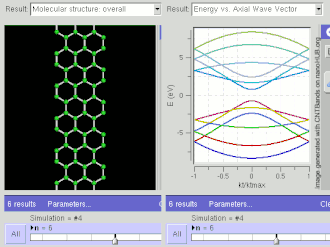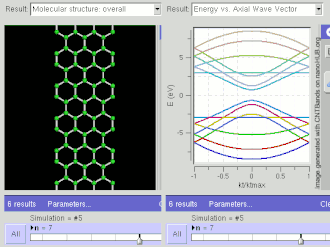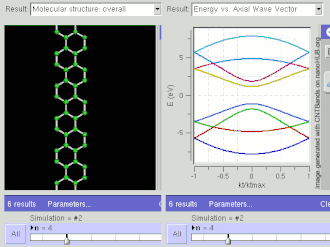 GNR band structure for armchair type. Tight binding calculations show that armchair type can be semiconducting or metallic depending on width (chirality).
GNR band structure for armchair type. Tight binding calculations show that armchair type can be semiconducting or metallic depending on width (chirality).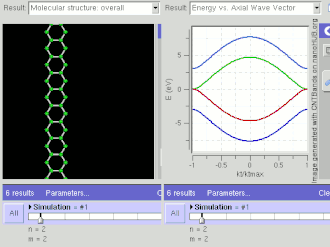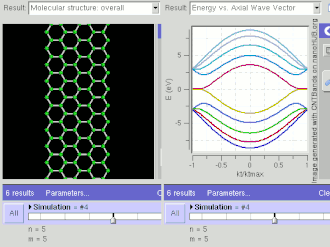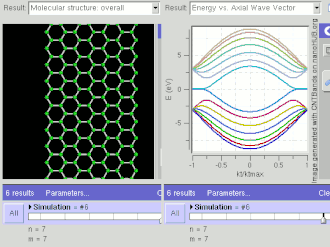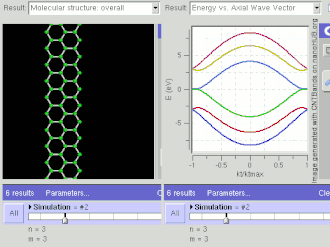 GNR band structure for zigzag type. Tight binding calculations predict that zigzag type is always metallic.
GNR band structure for zigzag type. Tight binding calculations predict that zigzag type is always metallic.
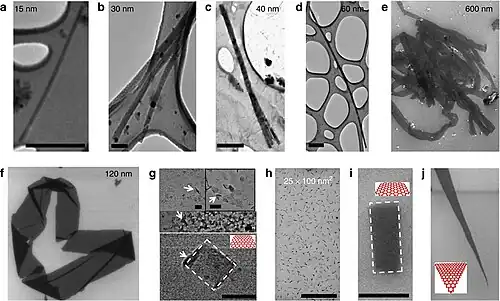 TEM micrographs of GNRs of (a) w=15, (b) w=30, (c) w=40 (exfoliating), and (d) w=60 nm deposited on 400 mesh lacey carbon grids and (e) FESEM micrograph of 600 nm ribbon. (f) Electron microscope images of a 120-nm graphene ribbons (FESEM), (g) 50 nm square GQDs (FESEM), (h,i) 25×100 nm2 rectangular GQDs (FESEM), and (j) 8°-angled tapered GNR (or triangular GQD) (FESEM)). The large densities of square and rectangular GQDs (g) showed extensive folding (white arrows). Bar sizes=(a) 250 nm, (b,g,i) 50 nm, (c,d) 500 nm, and (h) 1 μm.[5]
TEM micrographs of GNRs of (a) w=15, (b) w=30, (c) w=40 (exfoliating), and (d) w=60 nm deposited on 400 mesh lacey carbon grids and (e) FESEM micrograph of 600 nm ribbon. (f) Electron microscope images of a 120-nm graphene ribbons (FESEM), (g) 50 nm square GQDs (FESEM), (h,i) 25×100 nm2 rectangular GQDs (FESEM), and (j) 8°-angled tapered GNR (or triangular GQD) (FESEM)). The large densities of square and rectangular GQDs (g) showed extensive folding (white arrows). Bar sizes=(a) 250 nm, (b,g,i) 50 nm, (c,d) 500 nm, and (h) 1 μm.[5]
Mechanical properties
While it is difficult to prepare graphene nanoribbons with precise geometry to conduct the real tensile test due to the limiting resolution in nanometer scale, the mechanical properties of the two most common graphene nanoribbons (zigzag and armchair) were investigated by computational modeling using density functional theory, molecular dynamics, and finite element method. Since the two-dimensional graphene sheet with strong bonding is known to be one of the stiffest materials, graphene nanoribbons Young's modulus also has a value of over 1 TPa.[32][33][34]
The Young's modulus, shear modulus and Poisson's ratio of graphene nanoribbons are different with varying sizes (with different length and width) and shapes. These mechanical properties are anisotropic and would usually be discussed in two in-plane directions, parallel and perpendicular to the one-dimensional periodic direction. Mechanical properties here will be a little bit different from the two-dimensional graphene sheets because of the distinct geometry, bond length, and bond strength particularly at the edge of graphene nanoribbons.[32] It is possible to tune these nanomechanical properties with further chemical doping to change the bonding environment at the edge of graphene nanoribbons.[33] While increasing the width of graphene nanoribbons, the mechanical properties will converge to the value measured on the graphene sheets.[32][33] One analysis predicted the high Young's modulus for armchair graphene nanoribbons to be around 1.24 TPa by the molecular dynamics method.[32] They also showed the nonlinear elastic behaviors with higher-order terms in the stress-strain curve. In the higher strain region, it would need even higher-order (>3) to fully describe the nonlinear behavior. Other scientists also reported the nonlinear elasticity by the finite element method, and found that Young's modulus, tensile strength, and ductility of armchair graphene nanoribbons are all greater than those of zigzag graphene nanoribbons.[35] Another report predicted the linear elasticity for the strain between -0.02 and 0.02 on the zigzag graphene nanoribbons by the density functional theory model.[33] Within the linear region, the electronic properties would be relatively stable under the slightly changing geometry. The energy gaps increase from -0.02 eV to 0.02 eV for the strain between -0.02 and 0.02, which provides the feasibilities for future engineering applications.
The tensile strength of the armchair graphene nanoribbons is 175 GPa with the great ductility of 30.26% fracture strain,[32] which shows the greater mechanical properties comparing to the value of 130 GPa and 25% experimentally measured on monolayer graphene.[36] As expected, graphene nanoribbons with smaller width would completely break down faster, since the ratio of the weaker edged bonds increased. While the tensile strain on graphene nanoribbons reached its maximum, C-C bonds would start to break and then formed much bigger rings to make materials weaker until fracture.[32]
Optical properties
The earliest numerical results on the optical properties of graphene nanoribbons were obtained by Lin and Shyu in 2000.[20][37] The different selection rules for optical transitions in graphene nanoribbons with armchair and zigzag edges were reported. These results were supplemented by a comparative study of zigzag nanoribbons with single wall armchair carbon nanotubes by Hsu and Reichl in 2007.[38] It was demonstrated that selection rules in zigzag ribbons are different from those in carbon nanotube and the eigenstates in zigzag ribbons can be classified as either symmetric or antisymmetric. Also, it was predicted that edge states should play an important role in the optical absorption of zigzag nanoribbons. Optical transitions between the edge and bulk states should enrich the low-energy region ( eV) of the absorption spectrum by strong absorption peaks. Analytical derivation of the numerically obtained selection rules was presented in 2011,.[39][40][20] The selection rule for the incident light polarized longitudinally to the zigzag ribbon axis is that is odd, where and number the energy bands, while for the perpendicular polarization is even. Intraband (intersubband) transitions between the conduction (valence) sub-bands are also allowed if is even.

For graphene nanoribbons with armchair edges the selection rule is . Similar to tubes transitions intersubband transitions are forbidden for armchair graphene nanoribbons. Despite different selection rules in single wall armchair carbon nanotubes and zigzag graphene nanoribbons a hidden correlation of the absorption peaks is predicted.[41] The correlation of the absorption peaks in tubes and ribbons should take place when the number of atoms in the tube unit cell is related to the number of atoms in the zigzag ribbon unit cell as follows: , which is so-called matching condition for the periodic and hard wall boundary conditions. These results obtained within the nearest-neighbor approximation of the tight-binding model have been corroborated with first principles density functional theory calculations taking into account exchange and correlation effects.[42]
First-principle calculations with quasiparticle corrections and many-body effects explored the electronic and optical properties of graphene-based materials.[43] With GW calculation, the properties of graphene-based materials are accurately investigated, including graphene nanoribbons,[44] edge and surface functionalized armchair graphene nanoribbons[45] and scaling properties in armchair graphene nanoribbons.[46]
Analyses
Graphene nanoribbons can be analyzed by scanning tunneling microscope, Raman spectroscopy,[47][48] infrared spectroscopy,[49][50][51] and X-ray photoelectron spectroscopy.[52] For example, out-of-plane bending vibration of one C-H on one benzene ring, called SOLO, which is similar to zigzag edge, on zigzag GNRs has been reported to appear at 899 cm−1, whereas that of two C-H on one benzene ring, called DUO, which is similar to armchair edge, on armchair GNRs has been reported to appear at 814 cm−1 as results of calculated IR spectra.[50] However, analyses of graphene nanoribbon on substrates are difficult using infrared spectroscopy even with a Reflection Absorption Spectrometry method. Thus, a large quantity of graphene nanoribbon is necessary for infrared spectroscopy analyses.
Reactivity
Zigzag edges are known to be more reactive than armchair edges, as observed in the dehydrogenation reactivities between the compound with zigzag edges (tetracene) and armchair edges (chrysene).[53] Also, zigzag edges tends to be more oxidized than armchair edges without gasification.[54] The zigzag edges with longer length can be more reactive as it can be seen from the dependence of the length of acenes on the reactivity.[55]
Applications
Polymeric nanocomposites
Graphene nanoribbons and their oxidized counterparts called graphene oxide nanoribbons have been investigated as nano-fillers to improve the mechanical properties of polymeric nanocomposites. Increases in the mechanical properties of epoxy composites on loading of graphene nanoribbons were observed.[56] An increase in the mechanical properties of biodegradable polymeric nanocomposites of poly(propylene fumarate) at low weight percentage was achieved by loading of oxidized graphene nanoribbons, fabricated for bone tissue engineering applications.[57]
Contrast agent for bioimaging
Hybrid imaging modalities, such as photoacoustic (PA) tomography (PAT) and thermoacoustic (TA) tomography (TAT) have been developed for bioimaging applications. PAT/TAT combines advantages of pure ultrasound and pure optical imaging/radio frequency (RF), providing good spatial resolution, great penetration depth and high soft-tissue contrast. GNR synthesized by unzipping single- and multi-walled carbon nanotubes have been reported as contrast agents for photoacoustic and thermoacoustic imaging and tomography.[58]
Catalysis
In catalysis, GNRs offer several advantageous features that make them attractive as catalysts or catalyst supports. Firstly, their high surface-to-volume ratio provides abundant active sites for catalytic reactions. This enhanced surface area enables efficient interaction with reactant molecules, leading to improved catalytic performance.[59]
Secondly, the edge structure of GNRs plays a crucial role in catalysis. The zigzag and armchair edges of GNRs possess distinctive electronic properties, making them suitable for specific reactions. For instance, the presence of unsaturated carbon atoms at the edges can serve as active sites for adsorption and reaction of various molecules.
Moreover, GNRs can be functionalized or doped with heteroatoms to tailor their catalytic properties further. Functionalization with specific groups or doping with elements like silicon,[60] nitrogen, boron,[61] or transition metals can introduce additional active sites or modify the electronic structure, allowing for selective catalytic transformations.[62]
See also
- Graphene oxide paper
- Katsunori Wakabayashi
- Silicene, which can also form nanoribbons
- Graphene electronics
- Graphene helix
References
- ↑ Kawai, Shigeki; Saito, Shohei; Osumi, Shinichiro; Yamaguchi, Shigehiro; Foster, Adam S.; Spijker, Peter; Meyer, Ernst (2015). "Atomically controlled substitutional boron-doping of graphene nanoribbons". Nature Communications. 6: 8098. Bibcode:2015NatCo...6.8098K. doi:10.1038/ncomms9098. PMC 4560828. PMID 26302943.
- ↑ Fujita M.; Wakabayashi K.; Nakada K.; Kusakabe K. (1996). "Peculiar Localized State at Zigzag Graphite Edge". Journal of the Physical Society of Japan. 65 (7): 1920. Bibcode:1996JPSJ...65.1920F. doi:10.1143/JPSJ.65.1920.
- ↑ Nakada K.; Fujita M.; Dresselhaus G.; Dresselhaus M.S. (1996). "Edge state in graphene ribbons: Nanometer size effect and edge shape dependence". Physical Review B. 54 (24): 17954–17961. Bibcode:1996PhRvB..5417954N. doi:10.1103/PhysRevB.54.17954. PMID 9985930.
- ↑ Wakabayashi K.; Fujita M.; Ajiki H.; Sigrist M. (1999). "Electronic and magnetic properties of nanographite ribbons". Physical Review B. 59 (12): 8271–8282. arXiv:cond-mat/9809260. Bibcode:1999PhRvB..59.8271W. doi:10.1103/PhysRevB.59.8271. S2CID 119523846.
- 1 2 Mohanty, Nihar; Moore, David; Xu, Zhiping; Sreeprasad, T.S.; Nagaraja, Ashvin; Rodriguez, Alfredo Alexander; Berry, Vikas (2012). "Nanotomy Based Production of Transferable and Dispersible Graphene-Nanostructures of Controlled Shape and Size" (PDF). Nature Communications. 3 (5): 844. Bibcode:2012NatCo...3E.844M. doi:10.1038/ncomms1834. PMID 22588306.
- ↑ Brumfiel, G. (2009). "Nanotubes cut to ribbons New techniques open up carbon tubes to create ribbons". Nature. doi:10.1038/news.2009.367.
- ↑ Kosynkin, Dmitry V.; Higginbotham, Amanda L.; Sinitskii, Alexander; Lomeda, Jay R.; Dimiev, Ayrat; Price, B. Katherine; Tour, James M. (2009). "Longitudinal unzipping of carbon nanotubes to form graphene nanoribbons". Nature. 458 (7240): 872–6. Bibcode:2009Natur.458..872K. doi:10.1038/nature07872. hdl:10044/1/4321. PMID 19370030. S2CID 2920478.
- ↑ Liying Jiao; Li Zhang; Xinran Wang; Georgi Diankov; Hongjie Dai (2009). "Narrow graphene nanoribbons from carbon nanotubes". Nature. 458 (7240): 877–80. Bibcode:2009Natur.458..877J. doi:10.1038/nature07919. PMID 19370031. S2CID 205216466.
- ↑ "Writing Graphene Circuitry With Ion 'Pens'". ScienceDaily. March 27, 2012. Retrieved 29 August 2012.
- ↑ "AIP's Physics News Highlights March 27, 2012". American Institute of Physics (AIP). 2012-03-28. Retrieved 29 August 2012.
- ↑ Tongay, S.; Lemaitre, M.; Fridmann, J.; Hebard, A. F.; Gila, B. P.; Appleton, B. R. (2012). "Drawing graphene nanoribbons on SiC by ion implantation". Appl. Phys. Lett. 100 (73501): 073501. Bibcode:2012ApPhL.100g3501T. doi:10.1063/1.3682479.
- ↑ "Writing graphene circuitry with ion 'pens'". American Institute of Physics. Nanowerk News. March 27, 2012. Retrieved 29 August 2012.
- ↑ "New form of graphene allows electrons to behave like photons". kurzweilai.net. February 6, 2014. Retrieved October 11, 2015.
- ↑ Orcutt, Mike (August 13, 2015). "New Technique Gives Graphene Transistors a Needed Edge | MIT Technology Review". MIT Technology Review. Retrieved 2015-10-11.
- ↑ "'Armchair nanoribbon' design makes graphene a wafer-scalable semiconductor | KurzweilAI". www.kurzweilai.net. August 19, 2015. Retrieved 2015-10-13.
- ↑ Chen, Lingxiu; He, Li; Wang, Huishan (2017). "Oriented graphene nanoribbons embedded in hexagonal boron nitride trenches". Nature Communications. 8: 14703. arXiv:1703.03145. Bibcode:2017NatCo...814703C. doi:10.1038/ncomms14703. PMC 5347129. PMID 28276532.
- ↑ Yang, X.; Dou, X.; Rouhanipour, A.; Zhi, L.; Räder, H. J.; Müllen, K. (2008). "Two-Dimensional Graphene Nanoribbons". Journal of the American Chemical Society. 130 (13): 4216–4217. doi:10.1021/ja710234t. PMID 18324813.
- ↑ Dössel, L.; Gherghel, L.; Feng, X.; Müllen, K. (2011). "Graphene Nanoribbons by Chemists: Nanometer-Sized, Soluble, and Defect-Free". Angewandte Chemie International Edition. 50 (11): 2540–3. doi:10.1002/anie.201006593. PMID 21370333. S2CID 31349898.
- 1 2 "the Foresight Institute » Blog » Cleanly placing atomically precise graphene nanoribbons". www.foresight.org. 23 January 2017. Retrieved 2017-02-15.
- 1 2 3 4 5 6 Chung, H. C.; Chang, C. P.; Lin, C. Y.; Lin, M. F. (2016). "Electronic and optical properties of graphene nanoribbons in external fields". Physical Chemistry Chemical Physics. 18 (11): 7573–7616. arXiv:1510.01889. Bibcode:2016PCCP...18.7573C. doi:10.1039/C5CP06533J. PMID 26744847. S2CID 35857980.
- ↑ Barone, V.; Hod, O.; Scuseria, G. E. (2006). "Electronic Structure and Stability of Semiconducting Graphene Nanoribbons". Nano Letters. 6 (12): 2748–54. Bibcode:2006NanoL...6.2748B. doi:10.1021/nl0617033. PMID 17163699.
- ↑ Han., M.Y.; Özyilmaz, B.; Zhang, Y.; Kim, P. (2007). "Energy Band-Gap Engineering of Graphene Nanoribbons". Physical Review Letters. 98 (20): 206805. arXiv:cond-mat/0702511. Bibcode:2007PhRvL..98t6805H. doi:10.1103/PhysRevLett.98.206805. PMID 17677729. S2CID 6309177.
- ↑ Tapasztó, Levente; Dobrik, Gergely; Lambin, Philippe; Biró, László P. (2008). "Tailoring the atomic structure of graphene nanoribbons by scanning tunnelling microscope lithography". Nature Nanotechnology. 3 (7): 397–401. arXiv:0806.1662. doi:10.1038/nnano.2008.149. PMID 18654562. S2CID 20231725.
- ↑ Son Y.-W.; Cohen M. L.; Louie S. G. (2006). "Energy Gaps in Graphene Nanoribbons". Physical Review Letters. 97 (21): 216803. arXiv:cond-mat/0611602. Bibcode:2006PhRvL..97u6803S. doi:10.1103/PhysRevLett.97.216803. PMID 17155765. S2CID 536865.
- ↑ Jung. J.; Pereg-Barnea T.; MacDonald A. H. (2009). "Theory of Interedge Superexchange in Zigzag Edge Magnetism". Physical Review Letters. 102 (22): 227205. arXiv:0812.1047. Bibcode:2009PhRvL.102v7205J. doi:10.1103/PhysRevLett.102.227205. PMID 19658901. S2CID 6539197.
- ↑ Huang, Liang Feng; Zhang, Guo Ren; Zheng, Xiao Hong; Gong, Peng Lai; Cao, Teng Fei; Zeng, Zhi (2013). "Understanding and tuning the quantum-confinement effect and edge magnetism in zigzag graphene nanoribbon". J. Phys.: Condens. Matter. 25 (5): 055304. Bibcode:2013JPCM...25e5304H. doi:10.1088/0953-8984/25/5/055304. PMID 23300171. S2CID 9252524.
- ↑ Wang, Z. F.; Shi, Q. W.; Li, Q.; Wang, X.; Hou, J. G.; Zheng, H.; Yao, Y.; Chen, J. (2007). "Z-shaped graphene nanoribbon quantum dot device". Applied Physics Letters. 91 (5): 053109. arXiv:0705.0023. Bibcode:2007ApPhL..91e3109W. doi:10.1063/1.2761266. S2CID 119244435.
- ↑ Bullis, Kevin (2008-01-28). "Graphene Transistors". Technology Review. Cambridge: MIT Technology Review, Inc. Retrieved 2008-02-18.
- ↑ Bullis, Kevin (2008-02-25). "TR10: Graphene Transistors". Technology Review. Cambridge: MIT Technology Review, Inc. Retrieved 2008-02-27.
- ↑ Wang, Xinran; Ouyang, Yijian; Li, Xiaolin; Wang, Hailiang; Guo, Jing; Dai, Hongjie (2008). "Room-Temperature All-Semiconducting Sub-10-nm Graphene Nanoribbon Field-Effect Transistors". Physical Review Letters. 100 (20): 206803. arXiv:0803.3464. Bibcode:2008PhRvL.100t6803W. doi:10.1103/PhysRevLett.100.206803. PMID 18518566. S2CID 12833620.
- ↑ Ballon, M. S. (2008-05-28). Carbon nanoribbons hold out possibility of smaller, speedier computer chips. Stanford Report
- 1 2 3 4 5 6 Bu, Hao; Chen, Yunfei; Zou, Min; Yi, Hong; Bi, Kedong; Ni, Zhonghua (22 July 2009). "Atomistic simulations of mechanical properties of graphene nanoribbons". Physics Letters A. 373 (37): 3359–3362. Bibcode:2009PhLA..373.3359B. doi:10.1016/j.physleta.2009.07.048.
- 1 2 3 4 Faccio, Ricardo; Denis, Pablo; Pardo, Helena; Goyenola, Cecilia; Mombru, Alvaro (19 June 2009). "Mechanical properties of graphene nanoribbons". Journal of Physics: Condensed Matter. 21 (28): 285304. arXiv:0905.1440. Bibcode:2009JPCM...21B5304F. doi:10.1088/0953-8984/21/28/285304. PMID 21828517. S2CID 5099613 – via IOPscience.
- ↑ Georgantzinos, S.K.; Giannopoulos, G.I.; Anifantis, N.K. (December 2010). "Numerical investigation of elastic mechanical properties of graphene structures". Materials & Design. 31 (10): 4646–4654. doi:10.1016/j.matdes.2010.05.036.
- ↑ Georgantzinos, S.K.; Giannopoulos, G.I.; Katsareas, D.E.; Kakavas, P.A.; Anifantis, N.K. (May 2011). "Size-dependent non-linear mechanical properties of graphene nanoribbons". Computational Materials Science. 50 (7): 2057–2062. doi:10.1016/j.commatsci.2011.02.008.
- ↑ Changgu, Lee; Wei, Xiaoding; Kysar, Jeffrey; Hone, James (18 Jul 2008). "Measurement of the Elastic Properties and Intrinsic Strength of Monolayer Graphene". Science. 321 (5887): 385–388. Bibcode:2008Sci...321..385L. doi:10.1126/science.1157996. PMID 18635798. S2CID 206512830.
- ↑ Lin, Ming-Fa; Shyu, Feng-Lin (2000). "Optical Properties of Nanographite Ribbons". J. Phys. Soc. Jpn. 69 (11): 3529. Bibcode:2000JPSJ...69.3529L. doi:10.1143/JPSJ.69.3529.
- ↑ Hsu, Han; Reichl, L. E. (2007). "Selection rule for the optical absorption of graphene nanoribbons". Phys. Rev. B. 76 (4): 045418. Bibcode:2007PhRvB..76d5418H. doi:10.1103/PhysRevB.76.045418.
- ↑ Chung, H. C.; Lee, M. H.; Chang, C. P.; Lin, M. F. (2011). "Exploration of edge-dependent optical selection rules for graphene nanoribbons". Optics Express. 19 (23): 23350–63. arXiv:1104.2688. Bibcode:2011OExpr..1923350C. doi:10.1364/OE.19.023350. PMID 22109212. S2CID 119190424.
- ↑ Sasaki, K.-I.; Kato, K.; Tokura, Y.; Oguri, K.; Sogawa, T. (2011). "Theory of optical transitions in graphene nanoribbons". Phys. Rev. B. 84 (8): 085458. arXiv:1107.0795. Bibcode:2011PhRvB..84h5458S. doi:10.1103/PhysRevB.84.085458. S2CID 119091338.
- ↑ Saroka, V. A.; Shuba, M. V.; Portnoi, M. E. (2017). "Optical selection rules of zigzag graphene nanoribbons". Phys. Rev. B. 95 (15): 155438. arXiv:1705.00757. Bibcode:2017PhRvB..95o5438S. doi:10.1103/PhysRevB.95.155438.
- ↑ Payod, R.B.; Grassano, D.; Santos, G.N.C.; Levshov, D.I.; Pulci, O.; Saroka, V. A. (2020). "2N+4-rule and an atlas of bulk optical resonances of zigzag graphene nanoribbons". Nat. Commun. 11 (1): 82. Bibcode:2020NatCo..11...82P. doi:10.1038/s41467-019-13728-8. PMC 6941967. PMID 31900390.
- ↑ Onida, Giovanni; Rubio, Angel (2002). "Electronic excitations: Density-functional versus many-body Green's-function approaches". Rev. Mod. Phys. 74 (2): 601. Bibcode:2002RvMP...74..601O. doi:10.1103/RevModPhys.74.601. hdl:10261/98472.
- ↑ Prezzi, Deborah; Varsano, Daniele; Ruini, Alice; Marini, Andrea; Molinari, Elisa (2008). "Optical properties of graphene nanoribbons: The role of many-body effects". Physical Review B. 77 (4): 041404. arXiv:0706.0916. Bibcode:2008PhRvB..77d1404P. doi:10.1103/PhysRevB.77.041404. S2CID 73518107.
Yang, Li; Cohen, Marvin L.; Louie, Steven G. (2007). "Excitonic Effects in the Optical Spectra of Graphene Nanoribbons". Nano Lett. 7 (10): 3112–5. arXiv:0707.2983. Bibcode:2007NanoL...7.3112Y. doi:10.1021/nl0716404. PMID 17824720. S2CID 16943236.
Yang, Li; Cohen, Marvin L.; Louie, Steven G. (2008). "Magnetic Edge-State Excitons in Zigzag Graphene Nanoribbons". Physical Review Letters. 101 (18): 186401. Bibcode:2008PhRvL.101r6401Y. doi:10.1103/PhysRevLett.101.186401. PMID 18999843. - ↑ Zhu, Xi; Su, Haibin (2010). "Excitons of Edge and Surface Functionalized Graphene Nanoribbons". J. Phys. Chem. C. 114 (41): 17257. doi:10.1021/jp102341b.
- ↑ Zhu, Xi; Su, Haibin (2011). "Scaling of Excitons in Graphene Nanoribbons with Armchair Shaped Edges". Journal of Physical Chemistry A. 115 (43): 11998–12003. Bibcode:2011JPCA..11511998Z. doi:10.1021/jp202787h. PMID 21939213.
- ↑ Cai, Jinming; Pascal Ruffieux; Rached Jaafar; Marco Bieri; et al. (22 July 2010). "Atomicallyprecisebottom-upfabricationofgraphene nanoribbons". Nature. 466 (7305): 470–473. Bibcode:2010Natur.466..470C. doi:10.1038/nature09211. hdl:11858/00-001M-0000-000F-72E7-F. PMID 20651687. S2CID 4422290.
- ↑ Kim, Jungpil; Lee, Nodo; Min, Young Hwan; Noh, Seokhwan; Kim, Nam-Koo; Jung, Seokwon; Joo, Minho; Yamada, Yasuhiro (2018-12-31). "Distinguishing Zigzag and Armchair Edges on Graphene Nanoribbons by X-ray Photoelectron and Raman Spectroscopies". ACS Omega. 3 (12): 17789–17796. doi:10.1021/acsomega.8b02744. ISSN 2470-1343. PMC 6643467. PMID 31458375.
- ↑ Sasaki, Tatsuya; Yasuhiro Yamada; Satoshi Sato (6 August 2018). "Quantitative analysis of zigzag and armchair edges on carbon materials with and without pentagons using infrared spectroscopy". Analytical Chemistry. 90 (18): 10724–10731. doi:10.1021/acs.analchem.8b00949. PMID 30079720. S2CID 51920955.
- 1 2 Yamada, Yasuhiro; Masaki, Shiori; Sato, Satoshi (2020-08-01). "Brominated positions on graphene nanoribbon analyzed by infrared spectroscopy". Journal of Materials Science. 55 (24): 10522–10542. Bibcode:2020JMatS..5510522Y. doi:10.1007/s10853-020-04786-1. ISSN 1573-4803. S2CID 218624238.
- ↑ Kanazawa, Shuhei; Yamada, Yasuhiro; Sato, Satoshi (2021-04-22). "Infrared spectroscopy of graphene nanoribbons and aromatic compounds with sp3C–H (methyl or methylene groups)". Journal of Materials Science. 56 (21): 12285–12314. Bibcode:2021JMatS..5612285K. doi:10.1007/s10853-021-06001-1. ISSN 1573-4803. S2CID 233355287.
- ↑ Kim, Jungpil; Lee, Nodo; Min, Young Hwan; Noh, Seokhwan; Kim, Nam-Koo; Jung, Seokwon; Joo, Minho; Yamada, Yasuhiro (2018-12-31). "Distinguishing Zigzag and Armchair Edges on Graphene Nanoribbons by X-ray Photoelectron and Raman Spectroscopies". ACS Omega. 3 (12): 17789–17796. doi:10.1021/acsomega.8b02744. ISSN 2470-1343. PMC 6643467. PMID 31458375.
- ↑ Yamada, Yasuhiro; Kawai, Miki; Yorimitsu, Hideki; Otsuka, Shinya; Takanashi, Motoharu; Sato, Satoshi (2018-11-28). "Carbon Materials with Zigzag and Armchair Edges". ACS Applied Materials & Interfaces. 10 (47): 40710–40739. doi:10.1021/acsami.8b11022. ISSN 1944-8244. PMID 30339344. S2CID 206490799.
- ↑ Yamada, Yasuhiro; Kawai, Miki; Yorimitsu, Hideki; Otsuka, Shinya; Takanashi, Motoharu; Sato, Satoshi (2018-11-28). "Carbon Materials with Zigzag and Armchair Edges". ACS Applied Materials & Interfaces. 10 (47): 40710–40739. doi:10.1021/acsami.8b11022. ISSN 1944-8244. PMID 30339344. S2CID 206490799.
- ↑ Zade, Sanjio S.; Bendikov, Michael (2012). "Reactivity of acenes: mechanisms and dependence on acene length". Journal of Physical Organic Chemistry. 25 (6): 452–461. doi:10.1002/poc.1941. ISSN 1099-1395.
- ↑ Raifee, Mohammad; Wei Lu; Abhay V. Thomas; Ardavan Zandiatashbar; Javad Rafiee; James M. Tour (16 November 2010). "Graphene nanoribbon composites". ACS Nano. 4 (12): 7415–7420. doi:10.1021/nn102529n. PMID 21080652.
- ↑ Lalwani, Gaurav; Allan M. Henslee; Behzad Farshid; Liangjun Lin; F. Kurtis Kasper; Yi-Xian Qin; Antonios G. Mikos; Balaji Sitharaman (2013). "Two-Dimensional Nanostructure-Reinforced Biodegradable Polymeric Nanocomposites for Bone Tissue Engineering". Biomacromolecules. 14 (3): 900–9. doi:10.1021/bm301995s. PMC 3601907. PMID 23405887.
- ↑ Lalwani, Gaurav; Xin Cai; Liming Nie; Lihong V. Wang; Balaji Sitharaman (December 2013). "Graphene-based contrast agents for photoacoustic and thermoacoustic tomography". Photoacoustics. 1 (3–4): 62–67. doi:10.1016/j.pacs.2013.10.001. PMC 3904379. PMID 24490141.Full Text PDF.
- ↑ Peng, Hongliang; Duan, Diancheng; Liu, Siyan; Liu, Jiaxi; Sun, Lixian; Huang, Pengru; Shao, Chunfeng; Zhang, Kexiang; Zhang, Huanzhi; Xue, Xiaogang; Xu, Fen; Zou, Yongjin; Liu, Yalin; Tian, Xinlong; Rosei, Federico (2021-12-07). "A graphene-like nanoribbon for efficient bifunctional electrocatalysts". Journal of Materials Chemistry A. 9 (47): 26688–26697. doi:10.1039/D1TA06078C. ISSN 2050-7496. S2CID 240714294.
- ↑ Chen, Qu; Robertson, Alex W.; He, Kuang; Gong, Chuncheng; Yoon, Euijoon; Kirkland, Angus I.; Lee, Gun-Do; Warner, Jamie H. (2016-01-26). "Elongated Silicon–Carbon Bonds at Graphene Edges". ACS Nano. 10 (1): 142–149. doi:10.1021/acsnano.5b06050. ISSN 1936-0851. PMID 26619146.
- ↑ Gong, Yongji; Fei, Huilong; Zou, Xiaolong; Zhou, Wu; Yang, Shubin; Ye, Gonglan; Liu, Zheng; Peng, Zhiwei; Lou, Jun; Vajtai, Robert; Yakobson, Boris I.; Tour, James M.; Ajayan, Pulickel M. (2015-02-24). "Boron- and Nitrogen-Substituted Graphene Nanoribbons as Efficient Catalysts for Oxygen Reduction Reaction". Chemistry of Materials. 27 (4): 1181–1186. doi:10.1021/cm5037502. ISSN 0897-4756. OSTI 1185918.
- ↑ Xavier, Neubi F.; Bauerfeldt, Glauco F.; Sacchi, Marco (2023-02-08). "First-Principles Microkinetic Modeling Unravelling the Performance of Edge-Decorated Nanocarbons for Hydrogen Production from Methane". ACS Applied Materials & Interfaces. 15 (5): 6951–6962. doi:10.1021/acsami.2c20937. ISSN 1944-8244. PMC 9923683. PMID 36700729.