Ellipsoidal inclusion in an infinite medium

Ellipsoidal eigenstrain inclusion

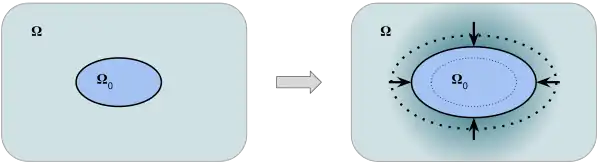
One of the earliest examples providing such a closed-form solution analyzed a ellipsoidal inclusion of material ${\displaystyle \Omega _{0}}$ with a uniform eigenstrain, constrained by an infinite medium ${\displaystyle \Omega }$ with the same elastic properties.^[6] This can be imagined with the figure on the right. The inner ellipse represents the region ${\displaystyle \Omega _{0}}$. The outer region represents the extent of ${\displaystyle \Omega _{0}}$ if it fully expanded to the eigenstrain without being constrained by the surrounding ${\displaystyle \Omega }$. Because the total strain, shown by the solid outlined ellipse, is the sum of the elastic and eigenstrains, it follows that in this example the elastic strain in the region ${\displaystyle \Omega _{0}}$ is negative, corresponding to a compression by ${\displaystyle \Omega }$ on the region ${\displaystyle \Omega _{0}}$.

The solutions for the total stress and strain within ${\displaystyle \Omega _{0}}$ are given by:

${\displaystyle \epsilon _{ij}=S_{ijkl}\epsilon _{kl}^{*}}$

${\displaystyle \sigma _{ij}=C_{ijkl}(\epsilon _{ij}-\epsilon _{kl}^{*})}$

Where ${\displaystyle S}$ is the Eshelby Tensor, whose value for each component is determined only by the geometry of the ellipsoid. The solution demonstrates that the total strain and stress state within the inclusion ${\displaystyle \Omega _{0}}$ are uniform. Outside of ${\displaystyle \Omega _{0}}$, the stress decays towards zero with increasing distance away from the inclusion. In the general case, the resulting stresses and strains may be asymmetric, and due to the asymmetry of ${\displaystyle S}$, the eigenstrain may not be coaxial with the total strain.

Inverse problem

Eigenstrains and the residual stresses that accompany them are difficult to measure (see:Residual stress). Engineers can usually only acquire partial information about the eigenstrain distribution in a material. Methods to fully map out the eigenstrain, called the inverse problem of eigenstrain, are an active area of research.^[5] Understanding the total residual stress state, based on knowledge of the eigenstrains, informs the design process in many fields.

Structural engineering

Residual stresses, e.g. introduced by manufacturing processes or by welding of structural members, reflect the eigenstrain state of the material.^[5] This can be unintentional or by design, e.g. shot peening. In either case, the final stress state can affect the fatigue, wear, and corrosion behavior of structural components.^[7] Eigenstrain analysis is one way to model these residual stresses.

Composite materials

Since composite materials have large variations in the thermal and mechanical properties of their components, eigenstrains are particularly relevant to their study. Local stresses and strains can cause decohesion between composite phases or cracking in the matrix. These may be driven by changes in temperature, moisture content, piezoelectric effects, or phase transformations. Particular solutions and approximations to the stress fields taking into account the periodic or statistical character of the composite material's eigenstrain have been developed.^[2]

Strain engineering

Lattice misfit strains are also a class of eigenstrains, caused by growing a crystal of one lattice parameter on top of a crystal with a different lattice parameter.^[8] Controlling these strains can improve the electronic properties of an epitaxially grown semiconductor.^[9] See: strain engineering.