hyperbolic geometry
English
Parallel lines in the Poincaré disk, a popular model of hyperbolic geometry. Many black lines, all parallel to the blue line, pass through the same point.
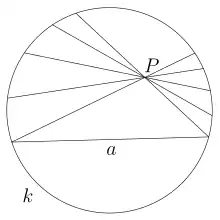
Multiple parallel lines through the point P, in the Beltrami–Klein model. Here, hyperbolic lines are Euclidean lines.
Etymology
The terminology was introduced in 1871 by Felix Klein, who classified some non-Euclidean geometries as hyperbolisch (“hyperbolic”), elliptisch (“elliptical”), and parabolisch (“parabolic”).
Noun
hyperbolic geometry (countable and uncountable, plural hyperbolic geometries)
- (geometry) A type of geometry that rejects the parallel postulate. Given a straight line L and a point P not on the line, more than one straight line can be drawn through P without intersecting L.
- (mathematics) A non-Euclidean geometry, that features the hyperbola as geodesic, and has constant negative curvature
Synonyms
- Lobachevsky-Bolyai-Gauss geometry
Further reading
- “hyperbolic geometry”, in Merriam-Webster Online Dictionary, Springfield, Mass.: Merriam-Webster, 1996–present.
hyperbolic geometry on Wikipedia.Wikipedia
This article is issued from Wiktionary. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.